QUESTION: How many ordinary sheets of paper would it take for every possible unique writing and drawing?
for every unique writing and drawing is
over 10 1,000,000 sheets of paper. The number looks like this:

Here are some examples and rules:
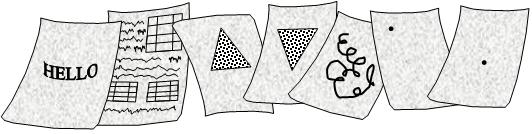
A sheet of paper is considered unique if any set of marks and/or non-marks (i.e., spaces), on that sheet does not match any other sheet.
The orientation of the sheet is kept constant so that similar objects skewed in different directions are also considered unique (see the two triangles above; one facing up and the other down).
The mark or marks made on the sheets can be as small as a pen or pencil point (a small finite size but not zero).
If the object appears exactly the same on different sheets yet are in different locations then the sheets are considered unique (see the two sheets with just one point on each).
If the exact same object (whether written and/or drawn), appears on several sheets with the only difference being one of size then the sheets are considered unique.
Two special sheets are also included: One is entirely blank (it's unique compared to all the other marked up sheets). The other is entirely filled in solid. Between the two are all the other sheets with varying degrees of markings and spaces.
[ Technical stuff that can be skipped. ]
To arrive at the mega-digit number given above it is assumed that the sheet of paper used is 8 by 11 inches and the smallest size of the marks on the page (pen or pencil tip), is 0.005 inches squared.
Using permutations with repetition, the calculation becomes,
A more precise value is 101,059,626 sheets of paper, or, the maximum number of writings and drawings, given the above conditions, that can be made on an ordinary sized sheet of paper.